Walkthrough, solutions, and answers for Pythagorea, a puzzle game in the Google Play and Apple iTunes store. This is the full solution, answer, and walkthrough for set 4, Medians and Midsegments. Follow along by downloading the app, and see the rest of the solutions at the bottom of this walkthrough!
For this walkthrough (solution, answer) for Pythagorea, the final solution will be shown. These can be seen as the final solution as the lines or dots are glowing orange.
Pythagorea Walkthrough Set 4, Medians and Midsegments
Level 1
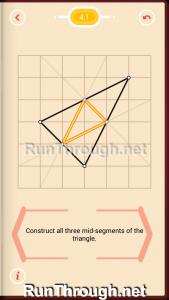
Level 2
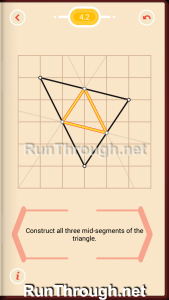
Level 3
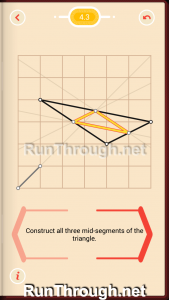
Level 4
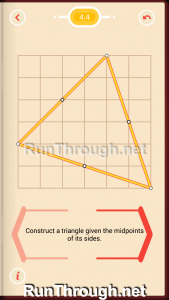
Level 5
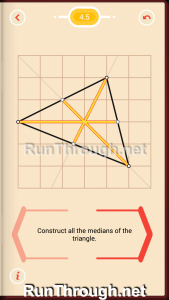
Level 6
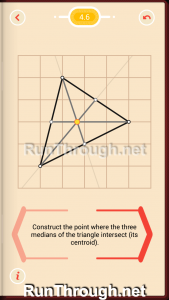
Level 7
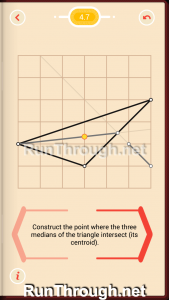
Level 8
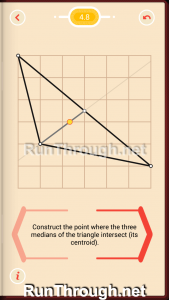
Level 9

Level 10

Level 11

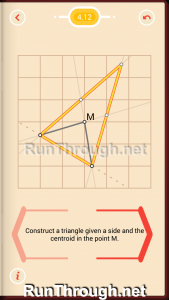
Level 12
Download: Google Play | iTunes
All Sets: 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 |19 | 20 | 21 | 22 | 23 | 24 | 25 | 26
See Pythagorea Review
Pythagorea Name Choice, Why Pythagorea
From the game’s iTunes and Google Play descriptions, the developer, Horis International Limited, says:
Pythagoras of Samos was a Greek philosopher and mathematician. He lived in 6th century BC. One of the most famous geometric facts bears his name: the Pythagorean Theorem. It states that in a right-angled triangle the area of the square on the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares of the other two sides. While playing Pythagorea you often meet right angles and rely on the Pythagorean Theorem to compare lengths of segments and distances between points. That is why the game is named after Pythagoras.
If you are interested in learning more about Pythagoras, please check out Wikipedia, or Math Open Reference (or just search his name of course!).
Enjoy playing Pythagorea, and if you ever need any help, pointers, or tips, keep coming back to this site and using our walkthrough, where we have every single answer and solution for the whole Pythagorea game. The links to all of the other set walkthroughs are above, and there is also a review linked to above.
Jul 25 2018
Pythagorea Set 4 Medians and Midsegments Walkthrough and Answers
Walkthrough, solutions, and answers for Pythagorea, a puzzle game in the Google Play and Apple iTunes store. This is the full solution, answer, and walkthrough for set 4, Medians and Midsegments. Follow along by downloading the app, and see the rest of the solutions at the bottom of this walkthrough!
For this walkthrough (solution, answer) for Pythagorea, the final solution will be shown. These can be seen as the final solution as the lines or dots are glowing orange.
Pythagorea Walkthrough Set 4, Medians and Midsegments
Level 1
Level 2
Level 3
Level 4
Level 5
Level 6
Level 7
Level 8
Level 9
Level 10
Level 11
Level 12
Download: Google Play | iTunes
All Sets: 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 |19 | 20 | 21 | 22 | 23 | 24 | 25 | 26
See Pythagorea Review
Pythagorea Name Choice, Why Pythagorea
From the game’s iTunes and Google Play descriptions, the developer, Horis International Limited, says:
If you are interested in learning more about Pythagoras, please check out Wikipedia, or Math Open Reference (or just search his name of course!).
Enjoy playing Pythagorea, and if you ever need any help, pointers, or tips, keep coming back to this site and using our walkthrough, where we have every single answer and solution for the whole Pythagorea game. The links to all of the other set walkthroughs are above, and there is also a review linked to above.
Related Posts:
By runner • Android, IOS, Mobile Games, Walkthroughs • • Tags: HORIS INTERNATIONAL LIMITED, puzzle