Walkthrough, solutions, and answers for Pythagorea, a puzzle game in the Google Play and Apple iTunes store. This is the full solution, answer, and walkthrough for set 2, Parallels. Follow along by downloading the app, and see the rest of the solutions at the bottom of this walkthrough!
For this walkthrough (solution, answer) for Pythagorea, the final solution will be shown. These can be seen as the final solution as the lines or dots are glowing orange.
Pythagorea Walkthrough Set 2, Parallels
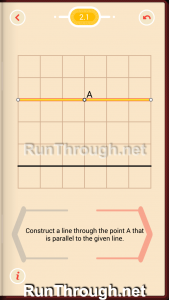
Level 1
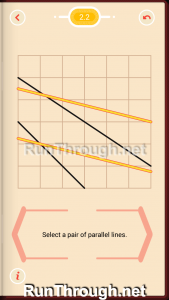
Level 2
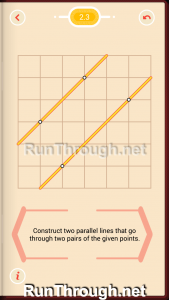
Level 3
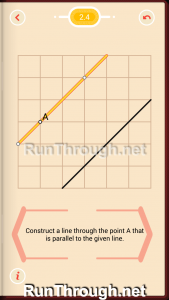
Level 4
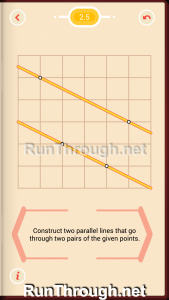
Level 5
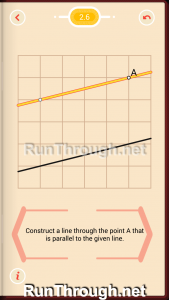
Level 6
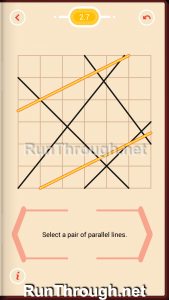
Level 7
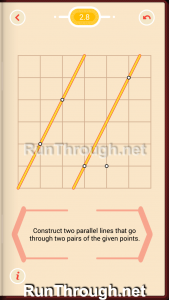
Level 8
Level 9
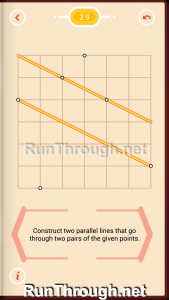
Level 10
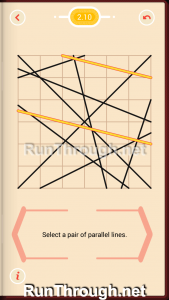
Level 11
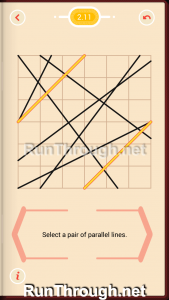
Level 12
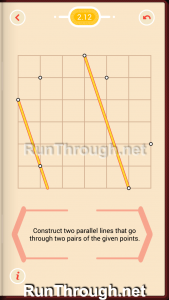
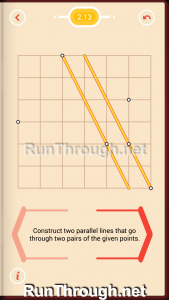
Level 13
Level 14

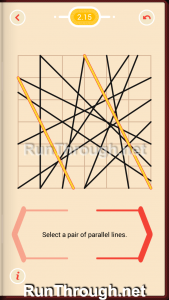
Level 15
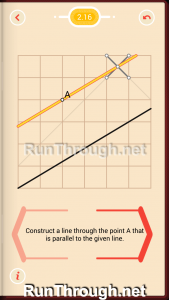
Level 16
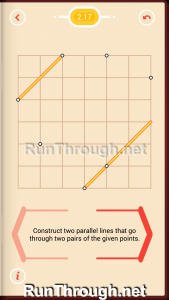
Level 17
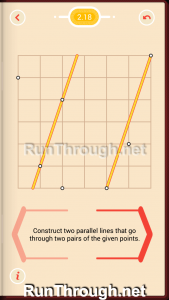
Level 18
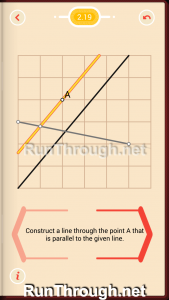
Level 19
Download: Google Play | iTunes
All Sets: 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 |19 | 20 | 21 | 22 | 23 | 24 | 25 | 26
See Pythagorea Review
Pythagorea Parallels/Parallel Lines Intro
Wikipedia gives the definition of parallel lines as “In geometry, parallel lines are lines in a plane which do not meet; that is, two lines in a plane that do not intersect or touch each other at any point are said to be parallel. By extension, a line and a plane, or two planes, in three-dimensional Euclidean space that do not share a point are said to be parallel. However, two lines in three-dimensional space which do not meet must be in a common plane to be considered parallel; otherwise they are called skew lines. Parallel planes are planes in the same three-dimensional space that never meet.” The photo below is a good example of what the section above is talking about:
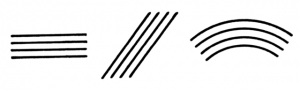
These lines are parallel, as they will never meet. As Pythagorea is exclusively in 2D, the only parallel you need to worry about is the lines, as planes will never come into play. Parallels is the second set of levels in Pythagorea, so these are also relatively easy, especially compared with some of the later levels, but there are always some levels that will stump someone, so that is why this walkthrough is here! All the answers and solutions for Pythagorea are on this site, so download to play along, and enjoy the game.
Jul 23 2018
Pythagorea Set 2 Parallels Walkthrough and Answers
Walkthrough, solutions, and answers for Pythagorea, a puzzle game in the Google Play and Apple iTunes store. This is the full solution, answer, and walkthrough for set 2, Parallels. Follow along by downloading the app, and see the rest of the solutions at the bottom of this walkthrough!
For this walkthrough (solution, answer) for Pythagorea, the final solution will be shown. These can be seen as the final solution as the lines or dots are glowing orange.
Pythagorea Walkthrough Set 2, Parallels
Level 1
Level 2
Level 3
Level 4
Level 5
Level 6
Level 7
Level 8
Level 9
Level 10
Level 11
Level 12
Level 13
Level 14
Level 15
Level 16
Level 17
Level 18
Level 19
Download: Google Play | iTunes
All Sets: 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 |19 | 20 | 21 | 22 | 23 | 24 | 25 | 26
See Pythagorea Review
Pythagorea Parallels/Parallel Lines Intro
Wikipedia gives the definition of parallel lines as “In geometry, parallel lines are lines in a plane which do not meet; that is, two lines in a plane that do not intersect or touch each other at any point are said to be parallel. By extension, a line and a plane, or two planes, in three-dimensional Euclidean space that do not share a point are said to be parallel. However, two lines in three-dimensional space which do not meet must be in a common plane to be considered parallel; otherwise they are called skew lines. Parallel planes are planes in the same three-dimensional space that never meet.” The photo below is a good example of what the section above is talking about:
These lines are parallel, as they will never meet. As Pythagorea is exclusively in 2D, the only parallel you need to worry about is the lines, as planes will never come into play. Parallels is the second set of levels in Pythagorea, so these are also relatively easy, especially compared with some of the later levels, but there are always some levels that will stump someone, so that is why this walkthrough is here! All the answers and solutions for Pythagorea are on this site, so download to play along, and enjoy the game.
Related Posts:
By runner • Android, IOS, Mobile Games, Walkthroughs • • Tags: HORIS INTERNATIONAL LIMITED, puzzle